sdhfnorm (Mu Analysis and Synthesis Toolbox)

Mu Analysis and Synthesis Toolbox

sdhfnorm

sdhfnorm calculates the induced norm of a sampled-data system

Syntax

```
[gaml,gamu]=sdhfsyn(p,k,h,delay,tol)
```

Description
sdhfnorm is concerned with the control of a continuous-time system by a discrete-time controller. The continuous-time interconnection structure structure, p of type SYSTEM, has state-space realization partitioned as follows:

where the continuous-time disturbance inputs enter through B₁, the outputs from the controller are held constant between sampling instants and enter through B₂, the continuous-time errors to be kept small correspond to the C₁ partition, and the output measurements that are sampled by the controller correspond to the C₂ partition. B₂ has column size (ncon) and C₂ has row size (nmeas). Note that the D matrix is assumed to be zero.

sdhfnorm calculates the maximum gain from the L₂ norm of the disturbance inputs to the L₂ norm of the error outputs.

Input arguments:

p
SYSTEM interconnection structure matrix, (continuous-time)

k
discrete-time controller

h
sampling period

delay
number of samples computational delay (default = 0)
(integer 0 with default =0)

tol
required relative accuracy

`p`	SYSTEM interconnection structure matrix, (continuous-time)
`k`	discrete-time controller
`h`	sampling period
`delay`	number of samples computational delay (default = 0) (integer 0 with default =0)
`tol`	required relative accuracy

Output arguments:

gaml
lower bound on the norm

gamu
upper bound on the norm

`gaml`	lower bound on the norm
`gamu`	upper bound on the norm

Examples
An illustrative example is given in the "Discrete-time and Sampled-data H· Control" section in Chapter 3.

Algorithm
sdhfnorm uses variations of the formulae described in the Bamieh and Pearson paper to obtain an equivalent discrete-time system. (These variations are done to improve the numerical conditioning of the algorithms.) A preliminary step is to determine whether the norm of the continuous-time system over one sampling period without control is less than the given 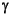-value. This requires a search and is, computationally, a relatively expensive step.

Subroutines called. dhfsyn, ham2schr, and compnorm

Reference
Bamieh, B.A., and J.B. Pearson, "A General Framework for Linear Periodic Systems with Applications to Sampled-Data Control," IEEE Transactions on Automatic Control, vol. AC-37, pp. 418--435, 1992.

See Also
dhfsyn, hinfsyne, hinffi, hinfnorm, hinfsyn, h2syn, h2norm, ric_eig, ric_schr

scliv sdhfsyn