

| Robust Control Toolbox |   |
Condition numbers of continuous algebraic Riccati equation.
Syntax
Description
Riccond provides the condition numbers of continuous Riccati equation. The input variable qrn contains the weighting matrix
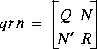
where P = P2/P1 is the positive definite solution of ARE, and [P2; P1] spans the stable eigenspace of the Hamiltonian
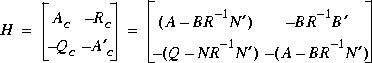
Several measurements are provided:
norAc, norQc, norRc of matrices Ac, Qc, and Rc, respectively.
conR of R.
conP1 of P1.
conArn) [1].
conBey) [2].
res).
tot puts the above measurements in a column vector
For an ill-conditioned problem, one or more of the above measurements could become large. Together, these measurements give a general sense of the Riccati problem conditioning issues.
Algorithm
Arnold and Laub's Riccati condition number is computed as follows [1]:
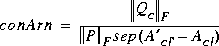
Byers' Riccati condition number is computed as [2]
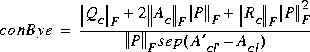
See Also
are, aresolv, daresolv, driccond
References
[1] W. F. Arnold, III and A. Laub, "Generalized Eigenproblem Algorithms and Software for Algebraic Riccati Equations," Proceedings of the IEEE, Vol. 72, No. 12, Dec. 1984.
[2] R. Byers, "Hamiltonian and Symplectic Algorithms for the Algebraic Riccati Equation," Ph.D. dissertation, Dept. of Comp. Sci., Cornell University, Ithaca, NY, 1983.
 | reig | sectf |  |