SimPowerSystems

Feedback Linearization Design

The input-output feedback linearization technique consists in the exact cancellation of the nonlinearities of the system in order to obtain a linear relationship between inputs and outputs in a closed loop. The nonlinear control law is deduced by successively differentiating each of the outputs until at least one input appears. Consider the first output as the terminal voltage V_t:

The terminal voltage is a complicated function of the state variables in which the control input V_fd appears explicitly with a multiplying factor of a very small order of magnitude. Ignore this direct dependence between V_t and V_fd and compute the derivative of output y₁.

The second output y₂ is the angle 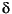that has to be differentiated three times before the inputs appear. This yields

Combining the equations of the outputs, the following input-output nonlinear system is obtained:

and the nonlinear control law is easily deduced.

This yields, in a closed loop, the exactly linearized input-output system

Once the system has been linearized, any linear control design can be applied to regulate the outputs. Here, the pole placement method was chosen and the following linear control law is proposed.

The last term in the equation of v₂ is introduced in order to stabilize the internal dynamics. These dynamics come about because the original nonlinear system is a ninth-order system while the linearized system is a fourth-order system. This is called partial linearization, and you must ensure that the remaining dynamics are asymptotically stable. A complete treatment of this question can be found in reference [1].

Mathematical Model

Simulation Results